A Day to Celebrate Fibonacci!

Fibonacci Day is an annual holiday celebrated on November 23 in honor of the mathematician Leonardo Bonacci commonly known as Fibonacci. Leonardo Bonacci is known for presenting the Fibonacci numbers as a way to popularize the Hindu–Arabic numeral system in the Western world.

Born in Pisa, present-day Italy 1175, Leonardo Bonacci was considered the brightest Western mathematician of the middle ages. With the Liber Abaci and his Fibonacci Sequence, he was later honored as Leonardo of Pisa by his city, and a statue of his likeness was erected there in the 19th century. As a child, Bonacci followed his merchant father to Algeria where he learned about the Hindu-Arabic numeral system.

“Fibonacci day itself, November 23rd, which can also be written as 11/23 in mm/dd format, is a Fibonacci sequence.”

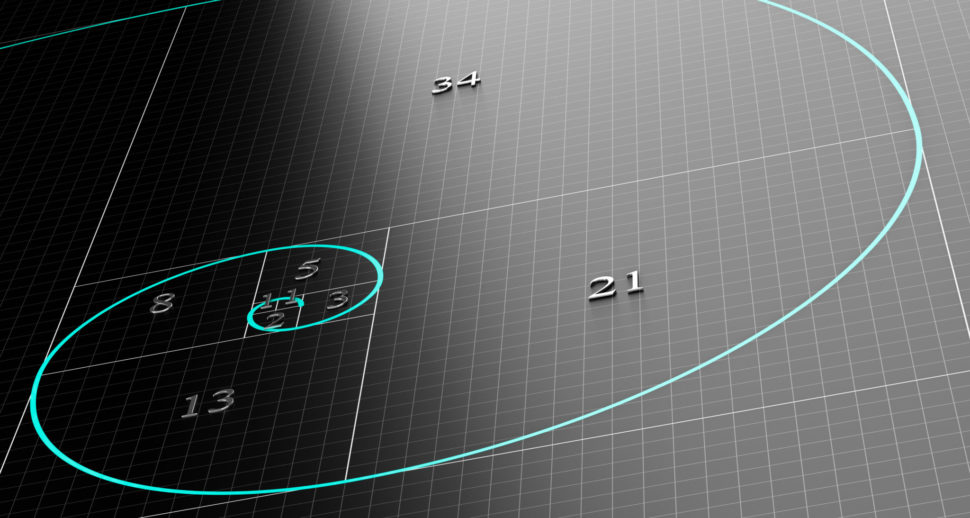
The Fibonacci Sequence

Each number in the Fibonacci sequence is the sum of the previous two numbers. An example sequence would be 1, 1, 2, 3, 5. Number 5, for instance, is the sum of 2 and 3, the preceding numbers.

The Fibonacci sequence was part of the Liber Abaci, Bonacci’s 1202 proposal to use the Hindu-Arabic numeral system, which he hoped would improve the western world’s ability to conduct business and keep records. The sequence itself was a solution to the mathematical problem of the growth of a population of rabbits. The proposed problem was what a rabbit population would grow to in one year starting with two newborn rabbits. Generation by generation, the idealized Fibonacci sequence successfully explained the rabbit’s population growth.

Fibonacci day itself, November 23rd, which can also be written as 11/23 in mm/dd format, is a Fibonacci sequence.

The Numbers in Nature

Fibonacci numbers are everywhere in the natural world, from a plant’s leaf organization, petals of the flower, to the arrangement of seeds on the flower head. Weather patterns leading to hurricanes and the pattern on a seashell also correspond to the sequence.

Even romanesco, a hybrid of broccoli and cauliflower, is a visual representation of nature’s correlation with the Fibonacci sequence.